Sumit Kumar Jha received his MS  Dual Degree in Computer Science and Engineering (CSE). His research work was supervised by Dr. Girish Varma. Here’s a summary of  Sumit Kumar Jha’s Partitions and Weighted Integer Compositions:

Suppose we wish to count the number of ways in which a positive integer n can be written as a sum of positive integers. If the order of the addends (or parts) matter, then the answer is

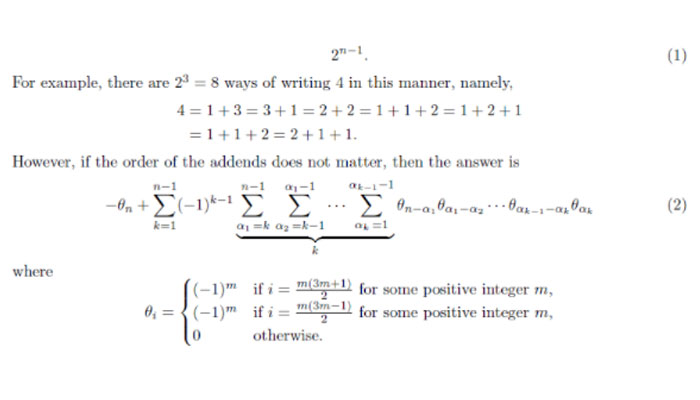
While (1) follows from a simple counting argument, a proof of (2) requires the use of generating functions and the theory of partial Bell polynomials.

We prove (2) in Chapter 2 using some tools developed in Chapter 1. This thesis concerns the subject of integer partitions. It has found numerous applications including in the study of black holes and in the area of statistical mechanics. A major area of investigation in its own right, it has been called \topic lled with the true romance of mathematics”.

Our investigation leads to following results in the subject:

• A combinatorial identity for the number of integer partitions of n in terms of certain weighted integer compositions of n.
• A combinatorial identity for the sum of positive divisors of n in terms of certain weighted integer compositions of n.

• A combinatorial identity for the number of representations of a positive integer n as a sum of k squares.

• A combinatorial identity for the number of representations of a positive integer n as a sum of k triangular numbers.

• A combinatorial identity for the number of plane partitions of a positive integer n.
• Some combinatorial identities for the Bernoulli numbers in terms of Stirling numbers of the second kind.

In deriving each of the above results, we highlight a useful combination of generating function techniques with the Faa di Bruno formula.